039 < >

On the Nature of Harmony

The first time I saw the overtone series my reaction was immediate. "Is this why people prefer major chords and other 'pleasant' harmonies and don't respond well to others? Is this what consonance and dissonance are all about?"

My presenter was a college-aged tutor at a summer music camp. She didn't know; probably hadn't thought about it. She took my question to the chairman of the theory department. I don't recall getting much of an answer from him, either. Maybe he just didn't want to open that can of worms.

Let me explain. Whenever you strike a note on a piano, or play one on a clarinet, you are not simply listening to that one note. There are countless other notes quietly humming about in different gradations of loud or soft, all of them below our threshold of conscious hearing. The relative strengths and weaknesses of the additional notes (called overtones, or partials) gives the sound its own particular character. This is why a clarinet sounds different from a piano. These partials are all pitched higher than the note you actually played, and they vibrate at the same distance from that note and from each other in the case of all musical instruments: in other words there is only one overtone series. If you are familiar with the piano, the following illustration will make sense to you. It shows the first 16 overtones in order:
  

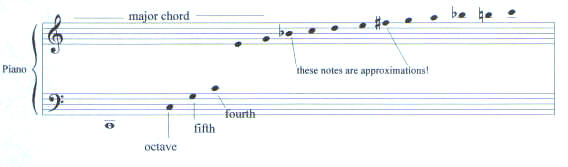If you played the first note, a low C, you would also get the rest of the notes vibrating quietly, even though they aren't loud enough to really notice. The first overtones tend to be strongest, and as the series ascends the partials get weaker. However, each instrument treats its overtones differently. In some, the odd-numbered partials are stronger, others have certain "favorites" that stick out above the others. These characteristic differences give each instrument a unique sound.

When I saw that chart I noticed immediately that the first four overtones make up a major chord. It made sense to me then that if that is the order in which musical tones vibrate in nature that that must be the reason our music is strewn with major chords; sounds we like, which sound complete and uncluttered to us.

A number of 20th century composer have noticed this as well, and some of them have used this phenomenon to suggest that music which is too harmonically complicated by their standards is not merely unpleasant, but even goes against nature itself. In other words, some people are going farther than people are supposed to go.

It would not be hard to make a case for why people tend to gravitate toward harmonies which replicate the overtone series; since they are hearing it all the time, at least subliminally, there must be a kind of magical 'yes' inside ourselves when it is presented to us audibly.

Then there is our inherent fondness for simplicity. The ancient Greek Pythagoras supposedly discovered that when you cut the length of a string in half and pluck it, the sound you get will be precisely one octave higher. This yields the simple ratio 2 to 1. It is not 17 to 15-and-a-half, there are no fractions here or complicated decimal places. It is simple. 2 to 1. The octave also happens to be the first member of the overtone series. For over a thousand years into the Christian era the only acceptable harmony was the octave (at least in church chant).

Eventually, however, man discovered that the fifth is also pleasant. If you took that same string and cut off a third of what remains, your note will sound a fifth higher (from c to g on the piano). The ratio is 3 to 2, also mystically simple. It captivated the medieval mind, as did the next in line, the ratio 4 to 3, which produces the fourth (c to f on the piano).

Then things get more complicated, numerically speaking. It was at this point that, until about the 15th century, and even to some degree after that, 'pleasing harmonies' stopped. Which is interesting, because we have, for those of you scoring at home, stopped just short of all the notes necessary to make up a major chord.

True enough, those sounds that have enchanted us for the past four centuries were once considered ugly and beyond the intention of creation. But eventually, musicians caved, and the modern 'chord' was born. Not that there wasn't some fierce debate over this.

If we follow that overtone series up its hazardous path, we see more notes that made their way into the pantheon of acceptable harmony as recently as the last century. The 7th was added to chords to give it four, not three members. Bach was already doing that. But keep adding notes, and jazz chords are soon called into existence. Again a furious debate in the musical community, social and musical.

Eventually one gets to the point where the notes get so close together that the piano can't play them. But before this happens there are notes which cause what most of us still feel is a harmonic clash with the first major chord. It is an e-flat, which is necessary in order to construct a minor chord. I have heard it said that nature is an optimist because it is the major and not the minor chord that is present in nature. But the minor is here as well, if quieter and higher up. In fact, both major and minor are always present at the same time, and we would hear them if we could hear the whole series of notes swirling about us. I bring all this up because I'd like to share something from the writings of one of America's most original composers who, one hundred years ago, was in the midst of his creativity, and whose thoughts were so far ahead of his time that he is hardly appreciated even today by laymen. He writes "They talk about some fundamental laws of sound--for instance, an obvious physical phenomenon, or rather a material arrangement of things, is 2 to 1 (that is, an octave). It happens to be self-evident, easy to hear and understand--but when you think of it, for that reason it is no more a fundamental law than 1 to 99....1 to 99 is just as fundamental and natural as 2 to 1. The physical movement of a string vibrating or dividing into segments is but a thing the eye and ear can know and see easily. Does that make it, or not make it, a fundamental law?"

"The obvious movements in the mechanico-physico world are too often by men taken for the whole, to a great extent, because it is easy to take them as such. Yet the overtones that a string may give are just as natural--more so-- than some of the triads used by the partialists as evidence of their fundamental laws."  (Ives, "Memos," p. 50) He goes on to point out that the way our pianos are tuned these days that the pleasant intervals we justify by the overtone series are, in fact, slightly off in many cases, which makes the idea of a simple "mirror of nature" seem absurd.

It seems to be no wonder that our harmonies would be dictated by natural phenomena, even one we can't actually hear. But while the overtone series recedes into complexity, so do we--at least, so have many of our musics. Even most pop music today makes use of notes that wouldn't have been in the vocabulary of the 18th century, to say nothing of more adventures styles. And yet, whenever there is an innovation, which often seems to heighten harmonic complexity, it is met with protests, and musicians of the 20th century often felt the need to discuss the matter in writing, something their predecessors in earlier centuries were unlikely to do. I leave you with the words of Ferucio Busoni, a pianist and composer whose music, though I know only a little of it, does not strike me as all that harmonically daring, relatively speaking. He explains the steady revealing of that tricky overtone series this way:

comments powered by Disqus